Introduction to Correcting Focus and Recompose
Chances are, you know what the “focus and recompose” technique is. But are you aware of its dangers as well?
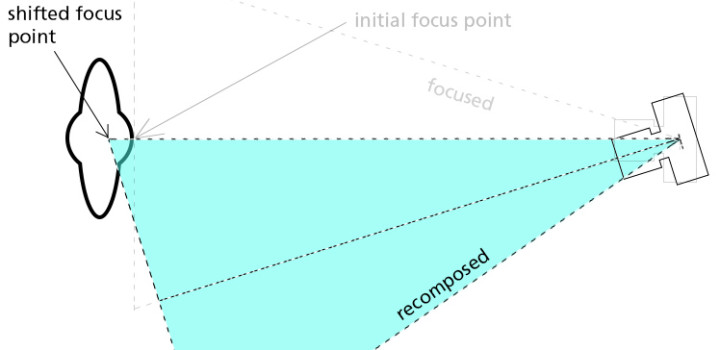
In a perfect world, the camera sensor, like our eye, would be curved, leading to a curved focal plane. Here, the focus and recompose technique WOULD work perfectly. However, since today’s camera sensors are flat, the focal plane will pan (or tilt) along with your recomposition which will lead to your subject moving outside the plane of focus. The following figure explains it more intuitively.
Traditional Solutions
Well there are two possibilities to cope with this focus problem:
- You could choose a focus point that is as close as possible to your subject in the recomposed field of view. Problem: Not all focus points are cross-type sensors, some of these border points will focus poorly in dim light.
- You could switch to live view and focus manually using focus peeking (digitally zoom in the live view to pixel level and then manually set your focus). Problem: a little slow and “finicky”; tripod probably a good idea.
Suggested Solution for Freaks
There is a third way and to my surprise it’s not much discussed. Probably because it involves a little bit of training and a fair bit of head calculation to get it down.
The focus shift depends on a couple of variables:
- focal length -> angle of view (degrees)
- distance to subject, that should be in focus
- amount of pan or tilt while recomposing
If we know these, we can calculate the focus error and compensate for it by stepping BACKWARDS accordingly.
What follows is a little bit of math, based on basic trigonometry. I suggest you skip it, if you’d just like to get to the application of this method.
Let’s assume your recompose is quite extreme, you’ll pan your camera to the right, so that the subject you initially focused on will be exactly on the image border.
In this case the error can be calculated as follows
For a 50mm lens (angle of view: 39.6º), at a distance of 100 cm, this would yield to a error of 6.283 cm. I completed a table for you with these. Let’s call them standard focus errors (at 1m distance).
| focal length (mm) | 16 | 20 | 24 | 35 | 50 | 70 | 85 | 105 | 200 |
| horizontal angle of view (º) | 95.1 | 82.4 | 73.7 | 54.4 | 39.6 | 28.8 | 23.9 | 19.5 | 10.3 |
| standard focus error (cm) | 48.160 | 32.905 | 24.967 | 12.433 | 6.283 | 3.244 | 2.214 | 1.456 | 0.405 |
You might have noticed the similarity to a depth of field chart for these lenses. And indeed the values are quite similar. For a 50mm at F/2.8 and 1 m focus distance, the DoP would as well be around 6 cm. However the similarity isn’t close enough to be used interchangeably. So don’t throw away that DoP chart yet – or rather that DoP app…
Practical application
Now that we know these standard focus errors. We can do a quick 2-step calculation in our heads. Let’s stick with the example of a 50mm lens.
Step 1
How far away is your subject. The standard focus error from the above table (in case you skipped the math part) is for 1 m distance. If your real distance is 3 m, you need to tripple that value
- 1 m standard distance for a 50mm lens: 6.283 cm
- 3 m actual distance: 3 x 6.283 cm = 18.849 cm
Step 2
How much did you recompose?
- If you recomposed the subject all the way to the border of the frame = 100% recompose = you will need to step backwards exactly the amount calculated in step 1 (18.849 cm)
- If you recomposed only 50% towards the border, then you only need to move back 50% of the value in step one: 50% of 18.849 = 9.434 cm
Tips
Since the standard focus errors stay constant for each lens, you could write it on a piece of tape and stick it on the lens itself. With time, you will remember the values and be much quicker with the calculation in your head. At least that’s what I hope for myself…
Final words
I imagine this must be more or less the way that Hasselblad does their TrueFocus system with their built-in accelerometer.
If the proposed method involves too much calculation for you, I don’t blame you! But just thinking about it might help to get a sense of when the traditional method using the center cross-type sensor is dangerous and when it can be safely used.
I’ll try to apply this technique in the following months and see what results it will yield and if, with practise, it can become faster and more reliable then the conventional techniques. I can imagine myself trying to apply it in situations, where I have a constant distance to the subject, e.g. for event or concert photography. Using prime lenses will simplify the process, because you only need to remember one value per lens. Let me know what you think in the comments.







Very interresting! I didn’t know this trick. BUT i have two questions :
1) The standard focus error you calculated is the same for full frame or crop sensor?
2) How do you do to know where exactly is 10 or 18 cm backward in space. I think it’s really difficult with my camera on the eye to know how much i have to go backward. AND my question is do i risk to have a worst result with my DOF if i go backward a little to much than if i just recompose?
You always learn me something, thank you!
Hi Christophe, the formula depends on the angle of view, which is smaller on the crop sensor camera. However, if you’d compare, say, a 55mm FF to a 35mm APS-C, the result would be the same, because the 35mm is ~55mm fullframe equivalent. But you definitely have different standard focus error values for the lenses on a APS-C.
your second question is a good one. You’re right that the DOF in front of the subject is often a little smaller then the one behind it, although not much. As long as you overstep the point suggested by the method AND not exit the DOF in front of the subject, you should be okay. This could become difficult, if your aperature is very wide (e.g. F/1.4) and your subject distance is close (e.g. macro shots). I’ll have to experiment with these situations to see, if my theory leeds to better focus or if I confuse things even more :).